
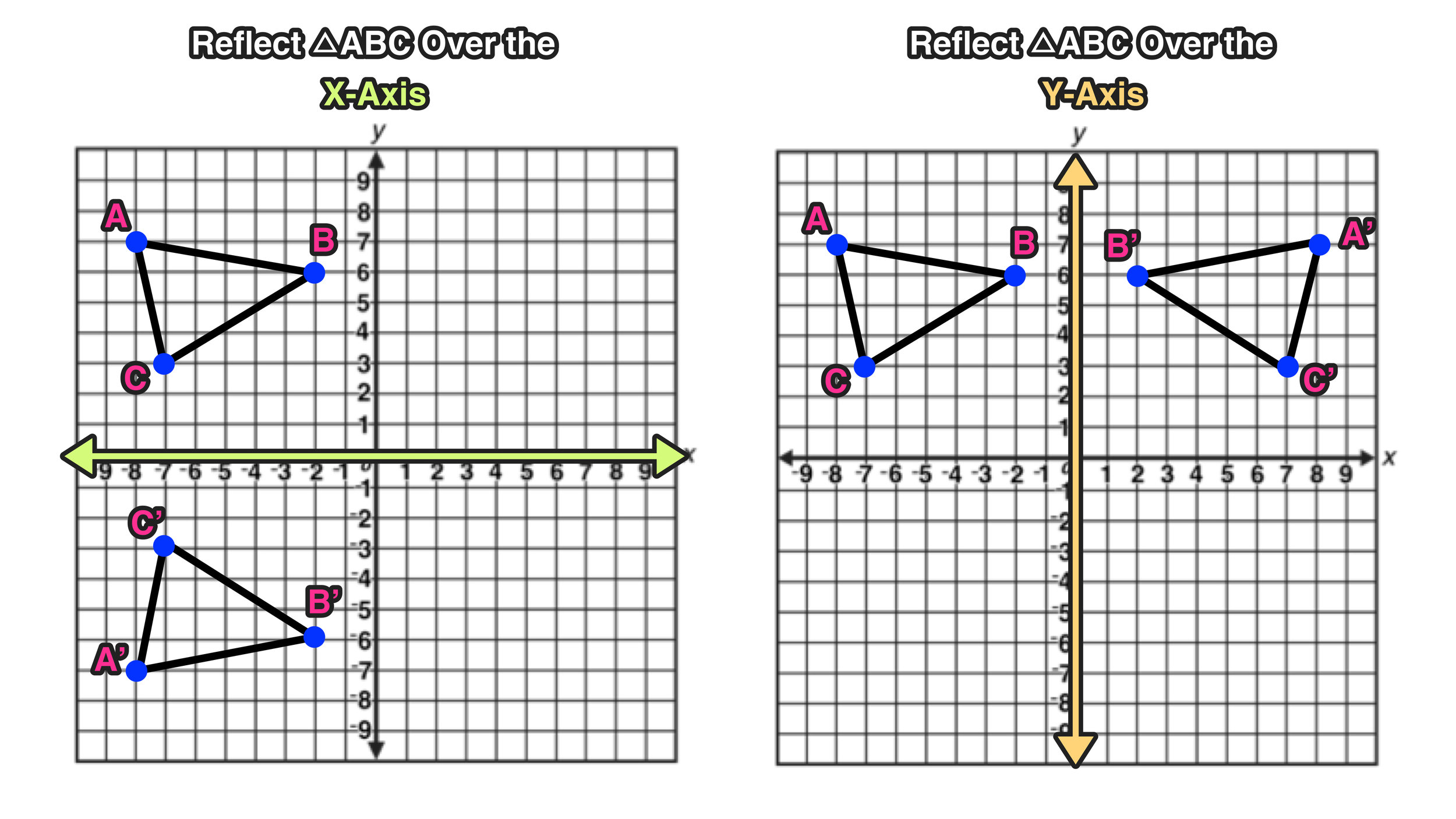
And then you can see that indeed do they indeed do look like reflections flipped over the X axis. And this bottom part of the quadrilateral gets reflected above it. Transformations Homework Packet Intro: Coordinate Plane Label the axes and origin reflection across the x-axis T(2. So you an kind of see this top part of the quadrilateral Ordered pair rules reflect over the x-axis: (x, -y), y-axis: (-x, y), line yx: (y, x). Corresponding parts of the figures are the same distance from the line of reflection. 3) reflection across the x-axis x y F G H 4) reflection across the y-axis x y N M L Find the coordinates of the vertices of each figure after the given transformation. What is the rule for reflection To perform a geometry reflection, a line of reflection is needed the resulting orientation of the two figures are opposite. Rewrite the transformation using reflection notation. And what's interesting about this example is that, the original quadrilateral is on top of the X axis. y Graph the image of the figure using the transformation given. We have constructed the reflection of ABCD across the X axis. And we'll keep our XĬoordinate of negative two. Unit below the X axis, we'll be one unit above the X axis. If we reflect across the X axis instead of being one If a reflection is about the y-axis, then, the points on the right side. even though rotating 90 degrees is not the same as reflecting about the y-axis,when you reflect again about the x-axis, it becomes the same as reflecting an. And so let's see, D right now is at negative two comma negative one. Besides translations, another kind of transformation of function is called reflection. Wiggle curve G8.04 Solving right triangles Chapter-35-1: Integral G11. So this goes to negative five, one, two, three, positive four. So it would have theĬoordinates negative five comma positive four. Units below the X axis, it will be four units above the X axis. The same X coordinate but instead of being four C, right here, has the X coordinate of negative five. The same X coordinate but it's gonna be two I'm having trouble putting the let's see if I move these other characters around. So let's make this right over here A, A prime. So, its image, A prime we could say, would be four units below the X axis.

So we're gonna reflect across the X axis. So let's just first reflect point let me move this a littleīit out of the way. Move this whole thing down here so that we can so that we can see what is going on a little bit clearer. So we can see the entire coordinate axis. An object and its reflection have the same shape and size, but. And we need to construct a reflection of triangle A, B, C, D. A reflection can be thought of as folding or flipping an object over the line of reflection. Tool here on Khan Academy where we can construct a quadrilateral.
In this case, theY axis would be called the axis of reflection.Asked to plot the image of quadrilateral ABCD so that's this blue quadrilateral here.
Math Definition: Reflection Over the Y AxisĪ reflection of a point, a line, or a figure in the Y axis involved reflecting the image over the Y axis to create a mirror image. Before diving deeper into the process of. Imagine a diagonal line passing through the origin, y x reflection occurs when a point or a given object is reflected over this line. In this case, the x axis would be called the axis of reflection. The y x reflection is a type of reflection on the Cartesian plane where the pre-image is reflected with respect to the line of reflection with an equation of y x. Pyramid, so that the y-axis passes through the vertex of the pyramid.
#Reflection over y axis how to
This complete guide to reflecting over the x axis and reflecting over the y axis will provide a step-by-step tutorial on how to perform these translations.įirst, let’s start with a reflection geometry definition: Math Definition: Reflection Over the X AxisĪ reflection of a point, a line, or a figure in the X axis involved reflecting the image over the x axis to create a mirror image. A coordinate grid is superimposed on a cross section of the Great. This idea of reflection correlating with a mirror image is similar in math. In real life, we think of a reflection as a mirror image, like when we look at own reflection in the mirror. Learning how to perform a reflection of a point, a line, or a figure across the x axis or across the y axis is an important skill that every geometry math student must learn.


 0 kommentar(er)
0 kommentar(er)
